Topic 2

Properties

There are four properties involving multiplication that will help make problems easier to solve. They are the commutative, associative, multiplicative identity and distributive properties.

Commutative property: When two numbers are multiplied together, the product is the same regardless of the order of the multiplicands. For example 4 * 2 = 2 * 4

Associative Property: When three or more numbers are multiplied, the product is the same regardless of the grouping of the factors. For example (2 * 3) * 4 = 2 * (3 * 4)

Multiplicative Identity Property: The product of any number and one is that number. For example 5 * 1 = 5.

Distributive property: The sum of two numbers times a third number is equal to the sum of each addend times the third number. For example 4 * (6 + 3) = 4*6 + 4*3

 

Practice Identifying them! - https://www.aaamath.com/pro74b-propertiesmult.html

Distributive Property Practice- https://www.ixl.com/math/grade-3/distributive-property-find-the-missing-factor

 

Greatest Common Factor

The greatest number that is a factor of two (or more) other numbers.

When we find all the factors of two or more numbers, and some factors are the same ("common"), then the largest of those common factors is the Greatest Common Factor.

Abbreviated "GCF". Also called "Highest Common Factor"

Example: the GCF of 12 and 16 is 4, because 1, 2 and 4 are common factors of both 12 and 16, and 4 is the greatest of them.

Practice:  https://www.ixl.com/math/grade-5/greatest-common-factor

 

*Know your Divisibility Rules! 

2	If the last digit is 0,2,4,6,8
3	If the SUM of the digits is divisible by 3  (add up the digits, can that number be divided by 3?)
4	If the last two digits of a number are divisible by 4.  (Example:  924.  The last two digits are 24.  24 is divisible by 4, so 924 is divisible by 4.)
5	If the last digit is a 5 or 0.
6	If the number is divisible by both 2 and 3.  (If you did the tests for 2 and 3…and they work, it’s also divisible by 6)
9	If the sum of the digits is divisible by 9.  (add the numbers up, is that number divisible by 9 ?)
10	If the last digit is 0.

 

 Topic 8

 https://quizlet.com/_588106 - vocabulary for topic 8.

 

 Lesson 1&2 Practice Quiz on Quizizz 

8-1

Things to know:

-Opposites- These are numbers the same distance from zero on a number line. 

Example:  The opposite of -4 is 4, the opposite of 9 is -9. 

 

 - You need to know examples of life situations that are represented by positive and negative numbers. 

Positive	Negative
25 degrees above zero	12 degrees below zero
Making $30 at work	Spending $15 on a toy
A mountain that is 1,000 feet above sea level	Land that is BELOW sea level
Gaining yards in a football game	Skiing down a mountain

 Topic 8 Lesson 1.pdf  -Lesson 1 worksheet. 

 

8-2

*Remember:  The larger a negative number, the LESS it's worth!  The further you go left on a number line, the smaller a number gets.  

 

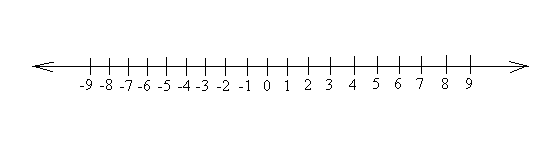
Helpful YouTube Video: 

 Comparing Integers game: http://www.sheppardsoftware.com/mathgames/integers/FS_CompareIntegers.htm

Ordering Integers game: https://www.mathplayground.com/number_climb.html

Homework Sheet : Topic 8 Lesson 2.pdf

 

8-3

 Absolute Value!  

 

 

 

 practice game:  http://www.xpmath.com/forums/arcade.php?do=play&gameid=96

 

 

 

8-4 Coordinate Planes 

 

Coordinate Plane game:  https://www.mathplayground.com/locate_aliens.html

Homework Sheet - Topic 8 Lesson 4.pdf

 

Make sure you understand this for the test!

 

 8-5 Distance 

 

*When finding distance, if you have two POSTIVE numbers, subtract them.

If you have one negative and one positive, add their absolute values.  

 

Example 1:  (3, 9) and (3,2).  The 3 is the same, so we find the distance of the "y".  There are two positive numbers, so we subtract 9 and 2.  The distance is 7.

 

Example 2:  (-6, -7)  and (3, -7).  The -7 is the same, so we find the distance of the "x".  There is one positive and one negative so we add their absolute values.

absolute value of -6 = 6

absolute value of 3 =3           6+3= 9